


Magnetic Fluids and Elastomers
related to DFG priority program SPP 1104 and SPP 1681
by H. Pleiner
(external collaboration with H.R. Brand)
In this project we want to derive the phenomenological theory of ferrofluids
that show additional macroscopic degrees of freedom like, e.g., ferronematics, ferrogels,
or ferrofluids with chaining in a strong external field.
This implies understanding and description of
the static and as well of the dynamic properties (e.g. flow behavior) of such
materials, the investigation of the influence of external (electric, magnetic,
or mechanical) fields, and the study of instabilities, the latter in
particular with respect to possible measurements of material parameters.
The existence of new macroscopic degrees of freedom gives rise to novel
effects, not possible in conventional ferrofluids. In addition, well-known
effects, which are small in other systems, can be enhanced considerably or
their threshold values can be reduced strongly in this unusual materials. This
can lead to new applications and/or to a better understanding even of
conventional ferrofluids.
Ferronematics I: We derive hydrodynamic-like equations to describe the static and dynamic macroscopic behavior of these materials. The macroscopic description of ferronematics differs from that of ordinary nematics in several ways. First, the magnetic susceptibility anisotropy is dramatically enhanced, thus allowing for a convenient orientation of ferronematics in external magnetic fields. In addition there are several dynamic crosscouplings, which are linear in the field. These effects are present in principle already in ordinary nematics, but generally neglected there, since they are assumed to be very small. In ferronematics, however, the response to external fields is very much enhanced and there is a good chance that they are observable. Examples are [C] reversible (Hall-like) heat, concentration and electric currents, reversible contributions to flow diffusion and director relaxation, as well as a dissipative analog of the flow alignment effect. We discuss, how these dynamic linear field effects can be measured directly. Since these measurements may be difficult to perform, we propose to measure the influence of the new effects on some standard instabilities, like Rayleigh-Benard and Saffmann-Taylor, where they lead to qualitatively new behavior [E]. For the former, with an external magnetic field parallel to the temperature gradient, we find above threshold a flow of vorticity along the field direction -- besides the usual convection rolls perpendicular to the field. This leads to an effective flow oblique to the rolls (in the homeotropic director orientation) and to oblique rolls (the roll axis is oblique to the director) for the planar case (the director fixed by an additional electric field). For the Saffmann-Taylor instability of a circular interface between air and the ferro-nematic liquid in a Hele-Shaw cell the evolving fingers rotate if a magnetic field perpendicular to the interface is applied. Since these effects are linear in the field, they will act in opposite direction when the field is reversed. This allows to discriminate them from those field effects due to the intrinsic field dependence of material parameters, since the latter is quadratic in the field and invariant under field reversal.
.
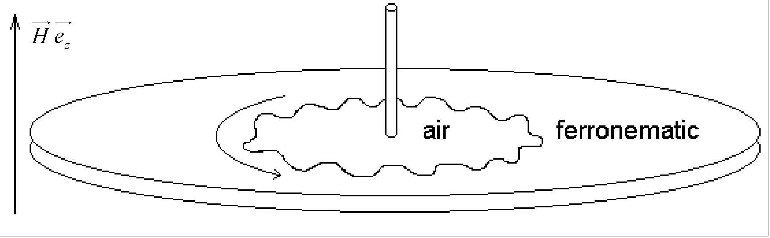
The setup of a radial Hele-Shaw cell. For a ferronematic
the external magnetic field leads to the rotation of the fingers shown by
the arrow.
Ferronematics II: On a third level of describing ferronematics suitable also for high frequencies the magnetization is taken into account as an independent degree of freedom. It is non-hydrodynamic in the superparamagnetic case (the truly ferromagnetic case has not been observed yet, although it is possible in principle [B]). Although magnetization and director are rigidly related to each other in equilibrium, this is not necessarily true in non-equilibrium situations. The standard hydrodynamic procedure reveals [D] that there is not only a static coupling between magnetization and director orientation, but also a dynamic one. In addition, there are reversible and irreversible couplings between flow and magnetization (and director rotations). Some of these couplings only exist, if an external field is applied (or if a spontaneous magnetization is present). They lead to qualitative changes in the sound spectrum and the rheological response to shear, in particular to a magnetic field dependence of sound damping and a coupling between sound and shear flow for almost all wave vector directions. We also investigate the response to an applied oscillating shear flow below the magnetic relaxation frequency. This allows to study the influence of the magnetic degree of freedom on the director dynamics. In an external field the absorption peak due to the director dynamics is shifted to a finite frequency that scales roughly with the third power of the field strength.
Ferrogels:
Ferrogels have been obtained by dissolving the magnetic particles in polymer solutions with subsequent crosslinking. They can be superparamagnetic and isotropic as well as anisotropic and having a frozen-in magnetization. Here, shape and volume changes induced by magnetic fields are of major interest for applications (artificial muscles).
In [F] we deal with the isotropic and paramagnetic ferrogels, only. In ferrogels the elastic degree of freedom takes over a role similar to the nematic one in ferronematics. The magnetoelasticity comes in the form of magnetostriction and through the magnetic part of the Maxwell stress and makes the system anisotropic in an external magnetic field. This gives rise to a field contribution of the sound spectrum at low frequencies that depends on the angle between field and wave vector. Various dynamic couplings of the elastic degree of freedom with the magnetization and flow are found. In the high frequency limit (above the magnetic relaxation frequency) the sound velocities are shifted due to those couplings. In addition we propose an experiment, where in a field gradient an oscillating temperature gradient produces a shear deformation perpendicular to both, the field and the temperature gradient.
A very specific way of investigating the dynamic interplay of elasticity and magnetic degree of freedom are surface waves. Here, apart from the usual capillary wave regime, an elastic wave regime is found. Applying a normal magnetic field to the free surface, surface waves break down and the Rosensweig instability develops above a critical threshold field. The latter increases with increasing elasticity, while the critical wavelength for the most unstable linear mode is independent of elasticity [H]. The resulting pattern of spikes has been analyzed using the somewhat heuristic energy method. Similar to the ferrofluid case, one-dimensional
stripe patterns are always unstable compared to two-dimensional square and hexagon patterns. The latter bifurcate subcritically, and then loose stability to squares well above the threshold. Both transitions are hysteretic, where the hysteresis range is diminished by elasticity [I].
Uniaxial magnetic gels are obtained by freezing-in a finite magnetization during crosslinking in the presence of an external field. The combination of a preferred direction, the magnetic degree of freedom, and elasticity makes this material unique and very peculiar [G]. Out-of-shear-plane flow alignment of the magnetization, enhanced momentum fluctuations due to a magnetization vorticity, and oscillating shear strains due to an oscillating external magnetic field are discussed.
Part of this text is based on "MACROSCOPIC DYNAMICS OF FERRONEMATICS AND FERROGELS" by E. Jarkova, A. Ryskin, H. Pleiner, and H.R. Brand in Proceedings Arbeitstagung Flüssigkristalle (Halle), 32, 24 (2004) [55 kB pdf-file]
Recent Publications:
H.R. Brand and H. Pleiner, "Origin of the Slow Wave in a Magneto-Rheological Slurry", [A]
Phys. Rev. Lett. 86, 1385 (2001) [28 kB ps.gz-file], [404 kB pdf-file] DOI: 10.1103/PhysRevLett.86.1385
H. Pleiner, E. Jarkova, H.-W. Müller, and H.R. Brand, "Landau description of ferrofluid to ferronematic phase transitions", [B1]
Magnetohydrodynamics 37, 254 (2001) [84 kB ps.gz-file], [794 kB pdf-file]
E. Jarkova, H. Pleiner, H.-W. Müller, A. Fink, and H.R. Brand, "Hydrodynamics of nematic ferrofluids", [C]
Eur. Phys. J. E 5, 583 (2001) [360 kB ps-file], [755 kB pdf-file]
E. Jarkova, H. Pleiner, H.-W. Müller, and H.R. Brand, "Macroscopic Dynamics of Ferronematics", [D]
J. Chem. Phys. 118, 2422 (2003) [258 kB ps.gz-file], [195 kB pdf-file] DOI: 10.1063/1.1533788
A. Ryskin, H. Pleiner, and H.-W. Müller, "Hydrodynamic instabilities in ferronematics", [E]
Eur. Phys. J. E 11, 389 (2003) [369 kB pdf-file] DOI: 10.1140/epje/i2003-10047-1
Proceedings Arbeitstagung Flüssigkristalle (Mainz) 31, P26 (2003) [124 kB pdf-file]
E. Jarkova, H. Pleiner, H.-W. Müller, and H.R. Brand, "Hydrodynamics of isotropic ferrogels", [F]
Phys. Rev. E 68, 041706 (2003) [207 kB pdf-file] DOI: 10.1103/PhysRevE.68.041706
S. Bohlius, H.R. Brand, and H. Pleiner, "Macroscopic dynamics of uniaxial magnetic gels", [G]
Phys. Rev. E 70, 061411 (2004) [227 kB pdf-file] DOI: 10.1103/PhysRevE.70.061411
S. Bohlius, H.R. Brand, and H. Pleiner, "Surface waves and Rosensweig instability in isotropic ferrogels", [H]
Z. Phys. Chem. 220, 97 (2006) [139 kB pdf-file] DOI: 10.1524/zpch.2006.220.1.97
S. Bohlius, H. Pleiner, and H.R. Brand, "Pattern Formation in Ferrogels: Analysis of the Rosensweig Instability Using the Energy Method", [I]
J. Phys.: Condens. Matter 18, S2671 (2006) [249 kB pdf-file] DOI: 10.1088/0953-8984/18/38/S10
S. Bohlius, H. Pleiner, and H.R. Brand, "Solution of the Adjoint Problem for Instabilities with a
Deformable Surface: Rosensweig and Marangoni Instability", [J]
Phys. Fluids 19, 094103 (2007) [241 kB pdf-file] DOI: 10.1063/1.2757709
S. Bohlius, H. Pleiner, and H.R. Brand, "Rosensweig Instability of Ferrogel Thin Films or Membranes", [K]
Eur. Phys. J. E 26, 275 (2008) [183 kB pdf-file] DOI: 10.1140/epje/i2007-10326-9
P. Martinoty, H.R. Brand, and H. Pleiner, "Physical Properties of Magnetic Gels" [M], in
Cross-Linked Liquid Crystalline Systems: From Rigid Polymer Networks to Elastomers, eds. G. Crawford, D. Broer, and S. Zumer (Taylor and Francis), Chapt. 18, p. 529-63 (2011)
[4.1 MB pdf-file] ISBN: 978-1-4200-4622-9.
H.R. Brand and H. Pleiner, "Macroscopic behavior of ferronematic gels and elastomers",
Eur. Phys. J. E 37, 122 (2014), [250 kB pdf-file] DOI: 10.1140/epje/i2014-14122-2.
H.R. Brand, A. Fink and H. Pleiner, "Macroscopic behavior of ferrocholesteric liquid crystals and ferrocholesteric gels and elastomers",
Eur. Phys. J. E 38, 65 (2015), [285 kB pdf-file] DOI: 10.1140/epje/i2015-15065-8.
T. Potisk, D. Svenšek, H.R. Brand, H. Pleiner, D. Lisjak, N. Osterman and A. Mertelj, "Dynamic Magneto-optic Coupling in a Ferromagnetic Nematic Liquid Crystal" ,
Phys. Rev. Lett. 119, 097802 (2017), DOI: 10.1103/PhysRevLett.119.097802
[1.5 MB pdf-file]
T. Potisk, A. Mertelj, N. Sebastián, N. Ostermann, D. Lisjak, H.R. Brand, H. Pleiner, and D. Svenšek, "Magneto-optic dynamics in a ferromagnetic nematic liquid crystal" ,
Phys. Rev. E 97, 012701 (2018), DOI: 10.1103/PhysRevE.97.012701
[5.4 MB pdf-file]
T. Potisk, H. Pleiner, D. Svenšek, and H.R. Brand, "Effects of flow on the dynamics of a ferromagnetic nematic liquid crystal",
Phys. Rev. E 97, 042705 (2018), [623 kB pdf-file] DOI: 10.1103/PhysRevE.97.042705.
T. Potisk, H. Pleiner, and H.R. Brand, "Dynamic interplay of nematic, magnetic, and tetrahedral order in ferromagnetic nematic phases",
Phys. Rev. E 98, 042703 (2018), [285 kB pdf-file] DOI: 10.1103/PhysRevE.98.042703.
T. Potisk, H. Pleiner, and H.R. Brand, "Influence of tetrahedral order on ferromagnetic gel phases",
Eur. Phys. J. E 42, 35 (2019), [330 kB pdf-file] DOI:10.1140/epje/i2019-11798-6.
H. Pleiner and H.R. Brand, "Symmetry aspects in the macroscopic dynamics of magnetorheological gels and general liquid crystalline magnetic elastomers",
Physical Sciences Reviews xx, 2019-0109 (2019), [448 kB pdf-file] DOI:10.1515/psr-2019-0109 -- or --
In Magnetic Hybrid-Materials, editor S. Odenbach, De Gruyter (2021), chapter 21, DOI: 10.1515.9783110569636 (ebook) and DOI: 10.1515.978311568776 (hard cover).
H. Pleiner and H.R. Brand, "Ferromagnetic nematics: A macroscopic two-fluid description",
J. Chem. Phys. 156, 204450 (2022), [4.6 MB pdf-file] DOI:10.1063/5.10080118.
preprints of the theory group
Other research topics:
Instabilities in Complex Fluids, Active Soft Matter
Banana and Tetrahedral Phases
Non-magnetic Liquid Crystalline Polymers and Elastomers
Membranes, Films and Surface Waves
General Mesophases
Rheology of Complex Fluids
Back to: 



HARALD PLEINER
Last modified: August 4th, 2006
Imprint(en): http://mpip-mainz.mpg.de/2908/imprint
Privacy Policy(en): http://mpip-mainz.mpg.de/347566/datenschutzhinweis





